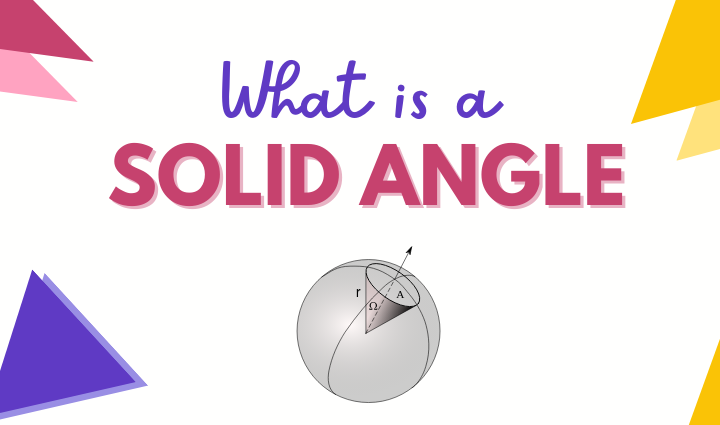
A solid angle is a fascinating concept in physics and geometry that extends the idea of a two-dimensional angle into three dimensions. By the end of this article, you’ll have a clear grasp of what a solid angle is, its formula, units, and practical examples. Let’s delve into this intriguing topic and discover its significance in various scientific fields.
What is a Solid Angle in Physics?
In physics, a solid angle is a measure of how large an object appears to an observer situated at a certain point. It is analogous to the two-dimensional angle but extends into three dimensions, providing a way to quantify how much of the space around a point is occupied by an object. Solid angles are crucial in fields such as astronomy, where they help describe how large celestial bodies appear from Earth.
Solid Angle Formula
The formula to calculate a solid angle is straightforward but essential for understanding its application:
Ω=Ar2\Omega = \frac{A}{r^2}
Where:
- Ω\Omega is the solid angle in steradians,
- AA is the area of the surface,
- rr is the radius from the point to the surface.
This formula shows that the solid angle depends on the area of the surface and the distance from the observer to the surface.
Solid Angle Calculator
A solid angle calculator can simplify the process of determining the solid angle subtended by an object. These tools require inputs such as the area of the object and the distance to the observer, then apply the formula to deliver an accurate measure of the solid angle in steradians. Such calculators are invaluable for students, engineers, and scientists who frequently work with solid angles.
Solid Angle Example
To illustrate, consider a cone. If the apex of the cone is at the origin and the base has a radius rr at a distance hh from the apex, the solid angle subtended by the base of the cone at the apex is given by:
Ω=2π(1−hh2+r2)\Omega = 2\pi \left(1 – \frac{h}{\sqrt{h^2 + r^2}}\right)
This example demonstrates the practical use of solid angles in understanding the spatial orientation of various shapes.
Solid Angle Unit
The unit of a solid angle is the steradian (sr). Just as the radian measures plane angles, the steradian measures solid angles in three-dimensional space. One steradian corresponds to the solid angle subtended by a unit sphere by a unit area on its surface.
Solid Angle of Sphere
A perfect example of a solid angle is the entire surface of a sphere. The solid angle subtended by a complete sphere from its center is 4π4\pi steradians. This is because the surface area of a sphere is 4πr24\pi r^2, and dividing this by r2r^2 gives 4π4\pi.
Solid Angle SI Unit
The SI unit of a solid angle is the steradian (sr). It is a dimensionless unit because it is derived from the ratio of the area to the square of the radius, which cancels out any dimensions.
Frequently Asked Questions (FAQs)
1. What is a solid angle in simple terms?
A solid angle is the three-dimensional equivalent of a two-dimensional angle, measuring how much of the space an object occupies as seen from a point.
2. How is a solid angle different from a plane angle?
While a plane angle measures the opening between two lines in a plane, a solid angle measures the size of the cone that an object forms at the viewpoint.
3. How do you measure a solid angle?
Solid angles are measured in steradians, using the formula Ω=Ar2\Omega = \frac{A}{r^2}, where AA is the area of the surface and rr is the distance from the point of observation.
4. Why are solid angles important?
Solid angles are crucial in fields such as physics, astronomy, and engineering, helping to quantify how large objects appear in three-dimensional space and understanding spatial relationships.
By understanding the concept of a solid angle, its calculation, and practical applications, you can appreciate its vital role in various scientific disciplines. Whether you’re observing the night sky or designing complex structures, the solid angle is a fundamental measure that helps bridge the gap between theoretical knowledge and practical application.